Net Present Value (NPV)
As you learned in the present value section, in order for you to compare the value of cash you receive or payout in the future, you need to convert those into a present value by discounting (taking out) all the compound interest effect so they become apple-to-apple, or comparable form. For example, you receiving $100 today and you receiving $100 5 years later aren’t comparable because they are on a different time horizon. So, you discounted $100 in Year 5 to its present value and derived $86.3. While the former (receive $100 today) remains as $100, because it’s already at the present value, the latter ($100 in Year 5) becomes $86.3. And now you know you are better off receiving $100 today rather than the same amount but 5 years later.
(If you don’t know what’s going on here, I strongly recommend you visit the present value section)
Now. You might wonder “what if you have more complex cash flow? If you have different cash flow with different amount and timing of cash receipt and cash payments, how can I evaluate and compare them?” This is where the Net Present Value, or NPV, kicks in.
“Net Present Value is the sum of discounted cash flows (=present values of future cash flow)”
As the name “net present value” suggests, all you need to do is simply (1) discount each years cash flow to present value and (2) add them all. The result will be the present value of all future cash flow combined.
Let’s see how it works.
Here are 2 cash flows: Cash Flow A and Cash Flow B.
Which cash flow do you think is beneficial for you? At a glance, Cash Flow A seems better off because if you add them all (add all cash receipt and subtract all cash payments), you end up with $282, while with Cash Flow B, you will have $268. The more, the better, right?
Well, not really. Why? If you thought Cash Flow A is worth $282 and Cash Flow B $268, you have totally ignored the time value of money concept, where compound interest effect affects the value of cash over time. For you to actually understand the value of each cash flow, you need to “discount” such effect of time and convert the cash flow to present values, make them apple-to-apple.
Let’s assume your discount rate is 5%. Then you need to discount cash flow of each year to the present value. Then, you add them all:
Do you see what happened here? While the simple addition of all cash flow resulted in $282 for Cash Flow A and $268 for Cash Flow B, the sum of the discounted cash flow (=sum of present values) for Cash Flow B ended up higher at $245, compared to Cash Flow A with $236. This indicates that given the timing of cash receipts and cash payments, Cash Flow B will end up more valuable than Cash Flow A. This is what the DCF does for you.
Now, this “sum of discounted cash flow”, or $236 for Cash Flow A and $24 for Cash Flow B, is called the Net Present Value. It’s, again, the net (=sum) of present values.
In this example, I’ve used the same time duration for both cash flow: 5 years. But, remember that NPV can even be calculated for different cash flow with different time range: You can compare one cash flow with 3 years duration and one with 10 years. Regardless of the time range, what NPV does remains the same: sums the present value of future cash flow, always making the outcome an apple-to-apple basis.
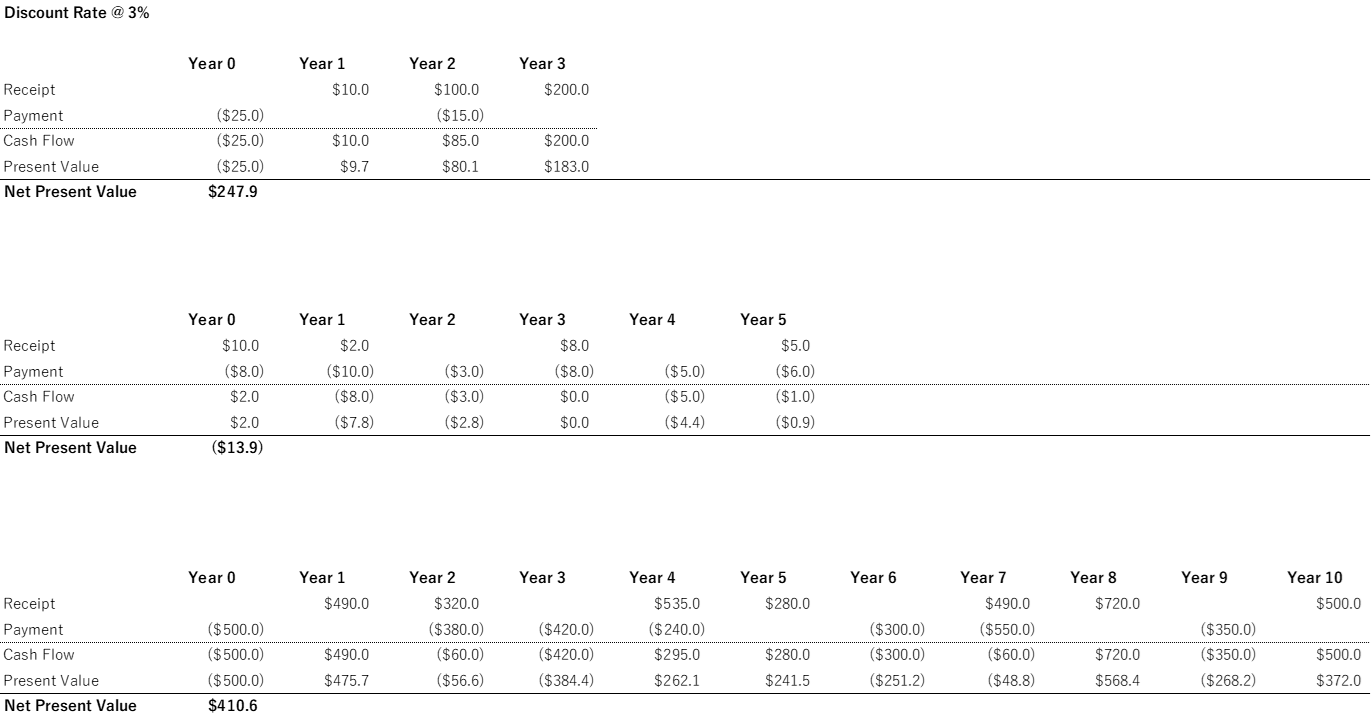
Below are another examples, but this time with 3% discount rate:
See?
Like I mentioned previously, NPV is the value of all the future cash flow. That means, if the NPV is positive, it indicates that the cash flow is profitable. When the NPV is negative, then the cash flow is not profitable.
Now, there’s one caveat: You should use the same discount rate when trying to compare different cash flows. If you use different discount rate for each cash flow, then the result is not truly an apple-to-apple. Yes, there could be cases where you need to analyze with different discount rate. Under such circumstance, make sure you write a footnote indicating so, otherwise it’ll be a misrepresentation.
This NPV exercise is quite fun. For you to get used to it, you should create your own cash flow with random numbers and see what NPV you can get. You should also try how the outcome changes if you change the discount rate. Because through the discounting, you are taking away the effect of compound interest effect. That means, if the discount rate becomes larger, you are taking away more compound interest effect, so the NPV will be smaller. By the same token, if the discount rate is smaller, you will have a larger NPV. Try it.
I hope now you have a good understanding of how NPV works.